8 Infinities on the Number Line

There are 8 distinct infinite boundaries that appear on the number line. Two either side of Zero, four that are found either side of the number +ONE and -ONE, and the final pair that is the infinite series of whole numbers, which increase towards infinity.
Overview
It is a mathematical fact that there are exactly eight infinities on the number line that divide positive from negative and whole and reciprocal numbers. By establishing the existence of the boundary of zero that manifests through infinite division, and the infinity of ONE that arises from reiterated root calculations, we can classify the number line into four distinct sections.
Through this, we can restructure the orientation of the number line into the quadratic nature of a square. This produces the foundations for a new mathematical view that supersedes our existing concept of the number i and the complex number plane.
KEy Points
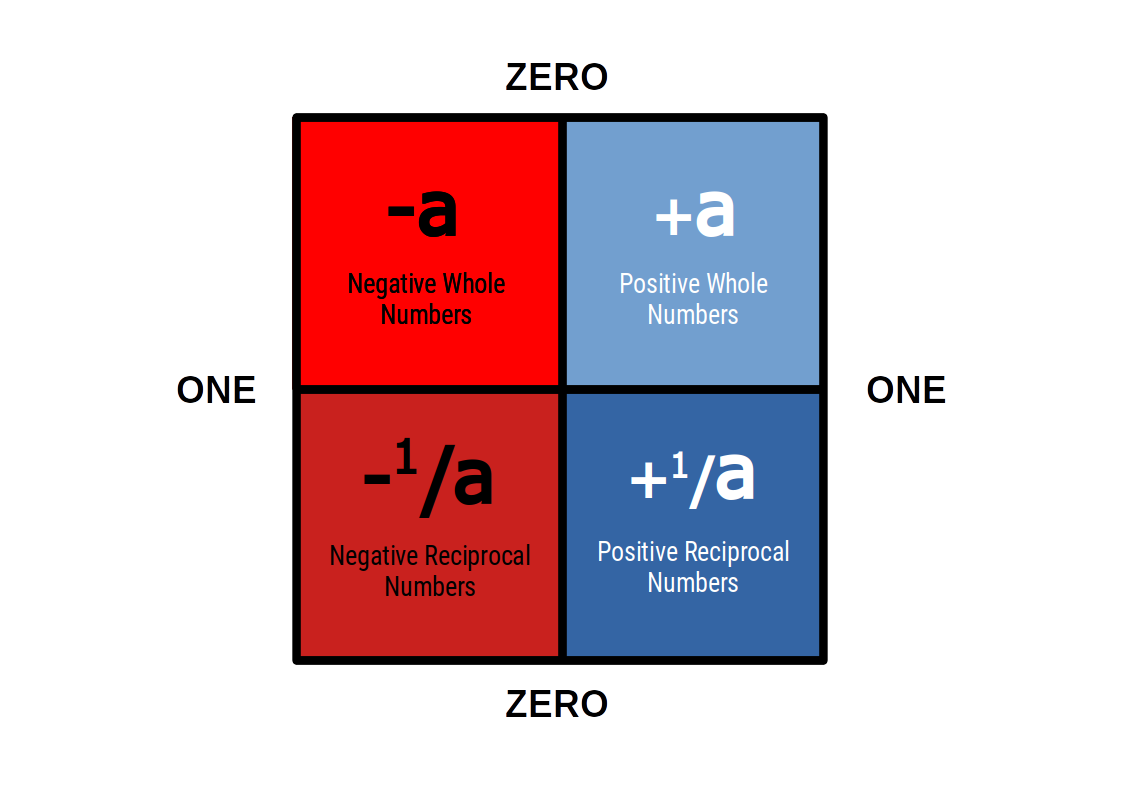
- There are four distinct sections on the number line. Positive and negative numbers, and their respective reciprocal spaces between zero and one.
- These sections are limited by 8 infinite boundaries, two either side of zero, two either side of -ONE and +ONE, and the last two derived from positive and negative whole numbers that exstend into infinity
- This establishes the quadratic nature of numbers, and allows us to reorientate the structure of the number line into a square, which radically shifts our understanding of the number ¡
THE
Concept
8 Infinities on the Number Line
The number line is the most familiar numerical construct used on earth today. Yet it appears that no one has taken the time to mathematically analyse its structure. By performing infinite recursive calculations, we find that the process of division reveals certain infinity boundaries. When any number is divided, it will reduce towards zero. Square root will diminish or increase towards ONE. And finally there are the two infinite set of whole numbers, positive and negative, that extend into infinity.
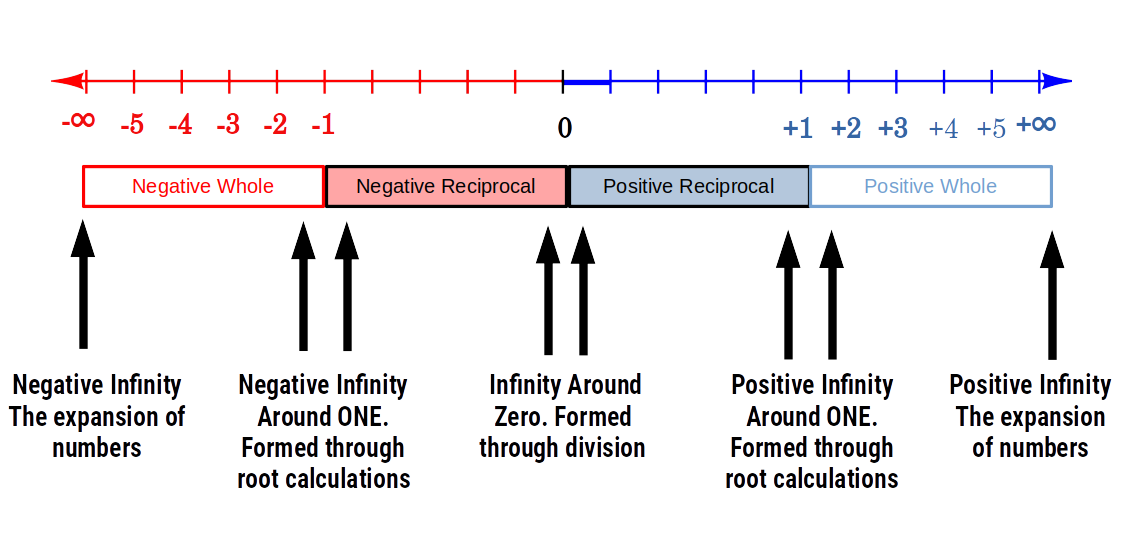
- Positive whole numbers extend into infinity when multiplied
- Negative whole numbers extend into infinity when mulitplied
- Positive numbers that diminish towards zero when divided
- Negative numbers that diminish towards zero when divided
- Positive whole numbers that move towards ONE when rooted
- Negative whole numbers that move towards ONE when rooted
- Positive reciprocal numbers that move towards ONE when rooted
- Negative reciprocal numbers that move towards ONE when rooted
Except for the first two, the other six boundaries can be found through the process of division. In the case of the infinite boundary around the number ONE, root calculations are reiterated, which is a function relating to the division of a quantity into equal parts.
Inverse to this is the function of squaring, or more precisely 'powers'. These are equal quantities are multiplied together. In the case of reciprocal values, the result will move towards zero, as if it were divided. This is because all the values are reciprocal numbers. It is the nature of multiplied reciprocals to therefore diminish towards zero, in the same way that their whole number counterpart will move further into infinity. This leads us to the realisation that the limiting boundary for the whole number series is in fact ZERO.
Watch the Video
We can demonstrate this structure of number using a simple Calculator. In this Video, Colin Power shows you how.
https://youtu.be/GPoEo9VCp1QREVIEW
Before reading on we recommend you check out these supporting posts, if you have not had the chance to do so already.
FIND OUT MORE ABOUT THE ZERO BOUNDARY
LEARN MOREFIND OUT MORE ABOUT THE INFINITY OF ONE
LEARN MOREFIND OUT MORE ABOUT RECIPROCAL SPACE
LEARN MORE4 types of number space
The discovery of these 8 types of infinite boundary allows us to deconstruct the number line into four types of infinite sets. These are:
- Positive whole numbers
- Negative whole numbers
- Positive reciprocal numbers
- Negative reciprocal numbers
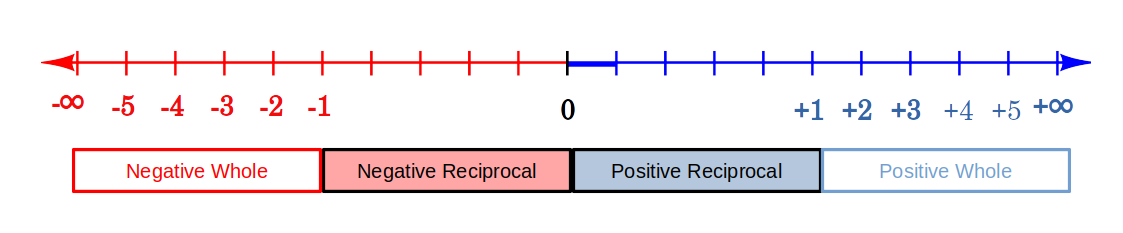
Each of these sections constitutes a unique infinite set. They each contain, not only all the whole numbers, but every fractional number too, although in reciprocal space whole numbers are also written as a fraction. With this realisation, we can begin to reorganise the traditional view of numbers into a geometric perspective, which helps us to make sense of the infinite qualities of numbers.
Key point
The number Line is composed of 4 types of infinite number sets
Reorientating the number line
The fact that the number line can be divided into four distinct parts, allows us to arrearage this traditional concept to reflect this quadratic nature.
Positive and negative
The first point of apparent symmetry on the number line is the infinite set of positive and negative numbers. Wherever there is a positive number, so we can find its negative 'reflection'.
Whether whole or reciprocal, both types can be written as either a negative or a positive value. In fact, it is the nature of negative and positive that allows us to perform addition and subtraction, if you think about it.

You sit at the Zero Point of your own reality
Whole and reciprocal
The second point of symmetry found on the number line is that of whole and reciprocal numbers. You can find out more about the details of reciprocal space in our post that covers the subject in more detail.
However, for now we can say that every number above ONE, including all fractions, has a reciprocal representation between zero and one.
In our post on the infinity of one, we explain why the numbers ZERO and ONE are 'special' numbers in that that form the infinite boundary that divides the number line into quadrants.

You sit at the Zero Point of your own reality
From these observations, we can arrange the number line into a square formation. The negative numbers are found on the left, whereas the positive are on the right. All whole numbers are contained in the two top squares, and all their reciprocal values in the bottom.

The traditional number line rearranged into a square to reflect
Notice that there is no longer any number line, instead we are representing the whole infinite set as contained within a square. This mathematical construction paves the way for a new kind of infinite maths, which is able to contain the entire infinite set of all positive, negative, whole (real), and reciprocal numbers.
However, we have also distinguished the number ONE and ZERO as being distinctly different from the rest. Just as a fence it held up by two posts, or a guitar string kept taut between the saddle and the far end of its fret board. So the numbers ZERO and ONE are boundaries that contain the infinite melodies of a universal orchestra of mathematics.
Solving Infinity
This simple geometric reordering of numbers actually has some quite profound solutions to the greatest mathematical problems of the 1900s. The Russell paradox was never resolved, and is key to the solution for the Continuum Hypothesis.
THE
Conclusion
What does this tell us about the number line?
From the boundaries creates by ZERO and ONE, the number line is divided into four distinct sections, form from eight types of infinite boundaries
It's not a line, it's a square?
Are the number line is quadratic in nature, it can be arranged into a square that can contain all real numbers, except for ONE and ZERO.
This new orientation holds deep implications for the mathematical concept of imaginary numbers.
Carry On Learning
This article is part of our new theory, 'Maths of Infinity'
Read the main article or browse more interesting post from the list below

YOUR QUESTIONS ANSWERED
Got a Question? Then leave a comment below.

Question?
So what about square and root numbers, where do they appear on your model?
ANSWER?
Actually as powers and roots are a mathematical function, the results form these equations are an expression of geometry itself. you can find out more about this in our post ZERO2.

Question?
So in the model, zero and one are not numbers? I thought that thery part of the set of whole numbers? After all doesn''t 0.5 x 2 = 1?
ANSWER?
This is that traditional view of numbers. However, as we have shown, due to the fact that ZERO and ONE exhibit infinite boundaries that no other number does, so we have developed a new class of number. One which is not presently defined. For this reason we often write the number 1 as ONE and 0 as ZERO when expressing this particular infinite quality.
STILL Looking for Answers?
Ask a question...
Comments
Post a Comment